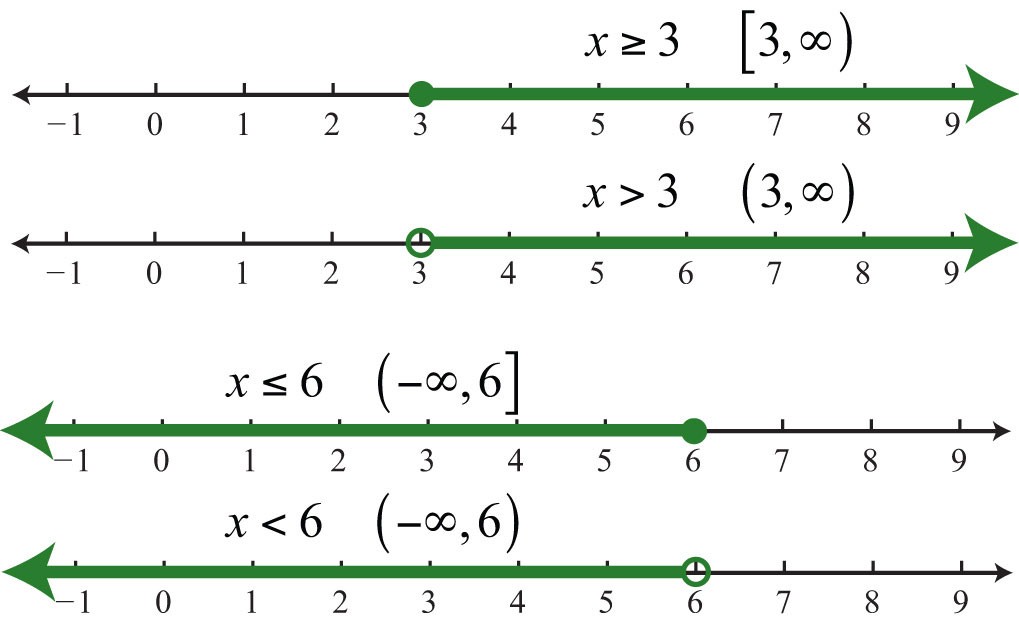
Inequalities are mathematical expressions used in a state where both sides are not equal to each other. Inequality can be either algebraic inequality or mathematical inequality, or a combination of both. Linear inequalities involve a linear algebraic expression where a polynomial of degree 1 gets compared with a polynomial of degree less than or equal to 1.
In general, there are seven means to solve linear inequalities in algebra. Keep reading to learn more about linear inequality. The mathematical tutorial answers questions like Keep reading to learn more about linear inequality. the mathematical tutorial answers questions like
- How can you graph a linear inequality?
- How can you solve linear inequality?
What are linear inequalities?
Linear inequalities compare two linear expressions using the symbols of inequality. Five linear expressions that are used for inequalities are as follows.
| Name of symbol | symbol | Example |
| Not equal | ≠ | y ≠ 4 |
| Greater than | > | 1+12 y > 2+14y |
| Less than | < | y+7 < √3 |
| greater than or equal to | ≥ | -4 – √4y ≥ 14 |
| less than or equal to | ≤ | y ≤ 5 |
One should note that if p < q, then p is a variable with a value less than q. It means that p is the variable that is less than q. It is the same for the other two inequalities. In a linear inequality like 3 y – 5 < 22.
In this case, LHS < RHS. One can easily understand that the Linear expression on the left-hand side of the equation is less than the right-hand side value. Visit Here: wpswebnews
All about the rules of linear inequalities
In general, one can perform four operations on linear expressions like subtraction, addition, division, and multiplication. Equivalent inequality refers to linear inequalities with the same solution. Both inequality and equality have distinct rules.
The same rule applies to Linear expressions with greater than equal to and lesser than equal to inequalities.
Addition rule of linear inequality
The addition law of linear inequality says that adding the same number on either side of the inequality leads to an equivalent inequality. It means that linear inequalities remain unaffected. It means that if m > n, Then m+x > n+ x. On the contrary, if m < n, then m+x < n+x.
Subtraction rule of linear inequality
The subtraction rule of linear inequalities says that subtracting the same value on either side of linear inequality leads to an equivalent inequality. In this case, linear inequalities remain unchanged. It means that if m > n Then m – x > n – x. On the contrary, if m < n then m – x < n – x.
A linear equation involving one variable
Let m and n be two non-zero real number and x be variable. Inequalities of the expression mx n > 0, mx n < 0 and mx n < 0 are linear inequalities having a single variable.
A linear equation involving two variables
Let m and n be two non-zero real numbers, y and x be two variables. Inequalities of the expression m+x+n+y < c, m+x+n+y > c are linear inequalities having two variables.
Note that solving multi-step linear equations is somewhat the same as solving multi-step linear inequalities.
Visit The Site: networldking52
It requires you to separate the variables from constants to solve a linear inequality. Don’t forget to inverse the sign of inequalities when dividing or multiplying the linear expressions with a negative integer.
Let’s take a look at the steps of solving linear expressions of inequalities.
Step 1: Simplify the inequalities on both sides, LHS and RHS.
Step 2: If it is a strict inequality, the value of x is less than or greater than defined in the equation. If it is not a strict inequality, the value for x is less than equal to or greater than equal to that defined in the equation.
Let’s try solving the algebraic equation. Only then you can grasp the concept.
Consider a linear equation where 3x 4 >10. Follow the steps to solve the linear inequalities.
3 x> 10 – 4 => 3x> 6 => x > 2
The solution of linear inequality will be the set of values of x that satisfy the equation x > 2. It holds for all the real values of x strictly greater than 2.
Let’s try solving the linear inequalities with variables on both sides. Follow the same concept that we applied before. For more clarity, let’s consider the equation as follows.
5x – 25 > 4x 11
Follow the steps to solve the linear inequality.
4x – 5 x > – 25 – 11
⇒ -x > -36
⇒ x > 36
Solving expressions of linear inequalities with graphical representation
Linear inequalities with two-variable expressions can take the form as follows. The signs of inequalities can change depending on the nature of expression. Note that to solve linear inequalities with two variables, we need two linear inequations.
Follow the steps to solve two linear inequalities with X and Y coordinates.
3 y – x > 2, or y – 3x < -2
- Students need to plot the linear inequalities in the graph. One needs to swap the inequality symbol with that of the equation. So, it becomes 3 y – x = 2, or y – 3x = -2. Since the inequality is strict, we draw dotted lines in the graph.
- Check whether the origin ( 0,0) satisfies the equation or not. If the answer is yes, get the side of the line shaded that includes the origin.
Otherwise, get the side of the line shaded that doesn’t include the origin.
For 3 y – x > 2, substitute the values of x and y.
It becomes 3 * 0 – 0 >2 => 0 > 2 which isn’t true. Shade the portion of the 3 y – x > 2, which doesn’t include origin. Similarly, for y – 3x < -2, we place ( 0,0) We have 0 -3 * 0< -2, which is not true as well. Shade the portion of the y – 3x < -2, which doesn’t include origin.
The common shaded portion in the chart is the clue to the linear inequality.
Mark the solution or simply shade it. If there is not a shaded area in the graph, the given linear inequalities do not exist.
Conclusion
To sum it up, you can easily solve any linear equation by supposing that the value of the variable is zero and then checking whether the inequality still exists.
Read More About: imnewsking